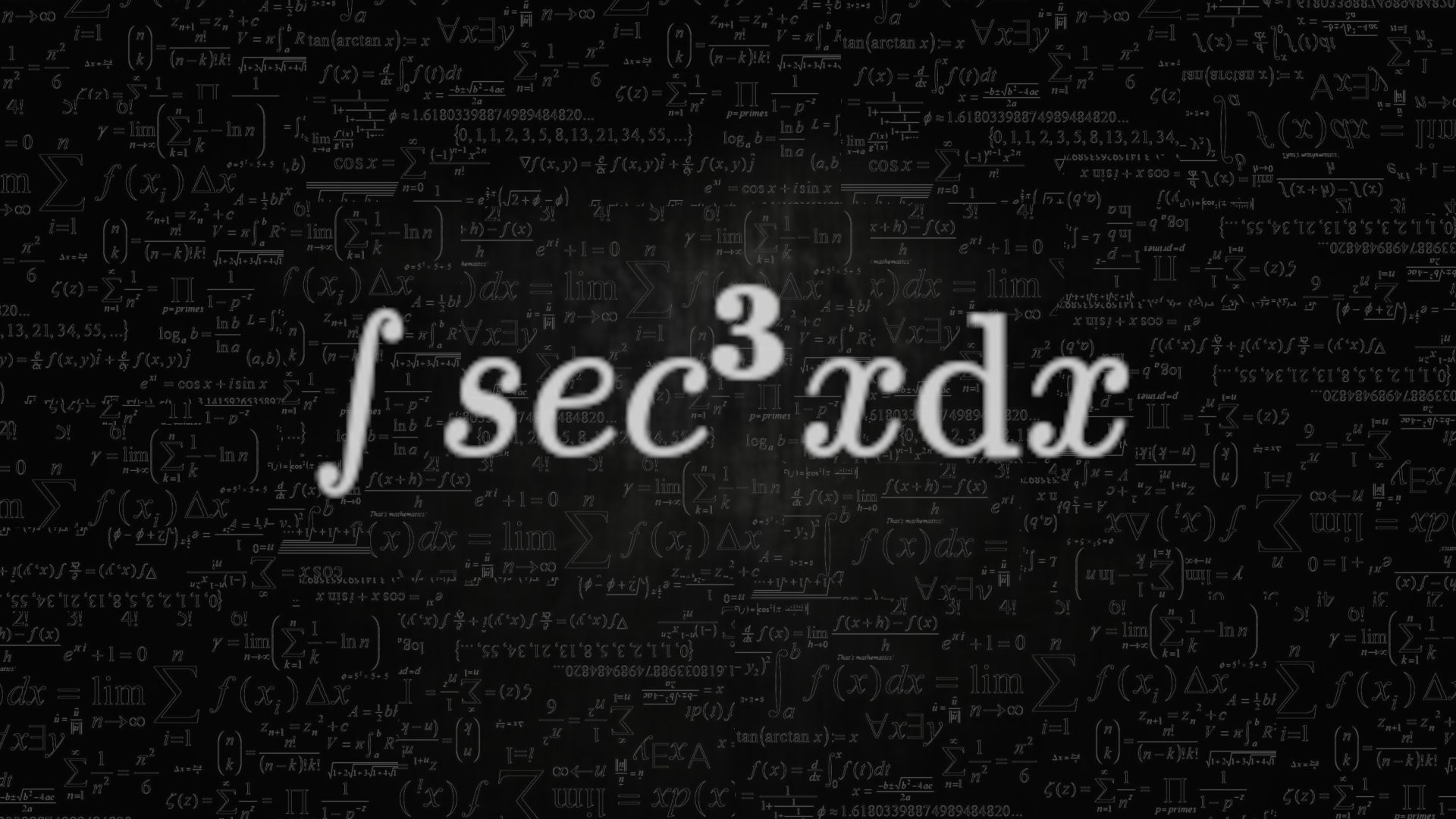How To Integrate sec^3(x)
Published On :2020-12-05 21:23:00
[begin{array}{l} smallint se{c^{bf{3}}}x{rm{d}}x\\ ;smallint secxse{c^2}x{rm{d}}x\ By;Parts\ Let Rightarrow u = sec x;;;;;;;;;;;;;;;;;;;;;;dv = se{c^2}x\ ;du = tan xsec xdx;;;;;;;;;;;;;;;;;;;v = tan x\ sec xtan x - smallint {tan ^2}xsec x{rm{d}}x\ sec xtan x - smallint sec xleft( {{{sec }^2}x - 1} right){rm{d}}x\ Rightarrow smallint {sec ^3}x{rm{d}}x = sec xtan x - smallint {sec ^3}x{rm{d}}x + smallint sec x{rm{d}}x\ Rightarrow 2smallint {sec ^3}{rm{d}}x = sec xtan x + ln left| {sec x + tan x} right| + c\ Rightarrow smallint {sec ^3}{rm{d}}x = frac{1}{2}(sec xtan x + ln left| {sec x + tan x} right|) + c end{array}]